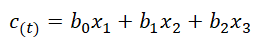El diagrama de Bode es el trazado de la respuesta de frecuencia de un sistema con gráficos de magnitud y fase separados. Las curvas de respuesta en frecuencia, de magnitud y de fase como funciones de log ω se denominan Diagramas de Bode. El dibujar diagramas de Bode se puede simplificar porque se pueden aproximar como una secuencia de líneas rectas. Las aproximaciones en línea recta simplifican la evaluación de la de magnitud y de fase de la respuesta en frecuencia.
Cuando elaboramos las gráficas de magnitud y de fase por separado, la gráfica de la curva de magnitud puede tener el eje de las ordenadas en decibeles (dB) vs. log ω en el eje de las abscisas, donde dB = 20 log M.
Ejemplo
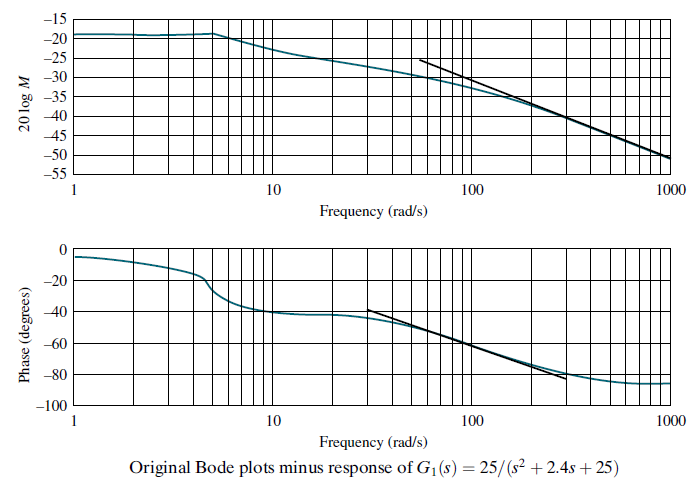
Graficar el Diagrama de Bode para la respuesta en frecuencia del sistema descrito por la función de Transferencia G(s):


Factores Básicos de G(jω)H(jω)
La ventaja principal de usar una traza logarítmica es la facilidad relativa de graficar las curvas de la respuesta en frecuencia. Los factores básicos que suelen ocurrir en una función de transferencia arbitraria G(jω)H(jω) son:
- La ganancia K
- Los factores de integral y de derivada
 ,
,
- Los factores de primer orden
 ,
,
- Los factores cuadráticos
 .
.
Una vez que nos familiarizamos con las trazas logarítmicas de estos factores básicos, es posible utilizarlas con el fin de construir una traza logarítmica compuesta para cualquier forma de G(jω)H(jω), trazando las curvas para cada factor y agregando curvas individuales en forma gráfica, ya que agregar los logaritmos de las ganancias corresponde a multiplicarlos entre sí.
El proceso de obtener la traza logarítmica se simplifica todavía más mediante aproximaciones asintóticas para las curvas de cada factor.
GANANCIA
La ganancia K. Un número mayor que la unidad tiene un valor positivo en decibeles, en tanto que un número menor que la unidad tiene un valor negativo.
La curva de magnitud logarítmica para una ganancia constante K es una recta horizontal cuya magnitud es de 20 log K decibeles. El ángulo de fase de la ganancia K es cero.

El efecto de variar la ganancia K en la función de transferencia es que sube o baja la curva de magnitud logarítmica de la función de transferencia en la cantidad constante correspondiente, pero no afecta la curva de fase.

POLOS Y CEROS EN EL ORIGEN
Factores de integral y de derivada (polos y ceros en el origen).
(polos y ceros en el origen).
Supongamos el factor 1/s en una función de transferencia cualquiera. El factor 1/s es un integrador. Es decir, la salida de ese sistema es la integral de la entrada. Al sustituir s=jω obtenemos el factor l/jω. La magnitud logarítmica de l/jω en decibeles es:

El ángulo de fase de l/jω es constante e igual a -90°.
En las trazas de Bode, las razones de frecuencia se expresan en términos de octavas o décadas. Una octava es una banda de frecuencia de ω1 a 2ω1, en donde ω1 es cualquier frecuencia. Una década es una banda de frecuencia de ω1 a 10ω1, en donde, otra vez, ω1 es cualquier frecuencia. (En la escala logarítmica del papel semi logarítmico, cualquier razón de frecuencia determinada se representa mediante la misma distancia horizontal. Por ejemplo, la distancia horizontal de ω=1 a ω=10 es igual a la de ω=3 a ω=30.
Si se gráfica la magnitud logarítmica de -20logω dB contra ω en una escala logarítmica, se obtiene una recta. Para trazar esta recta, necesitamos ubicar un punto (0 dB, ω= 1) en ella. Dado que:

La pendiente m de la recta para l/jω es de:

El ángulo de fase del factor l/jω es constante e igual a -90°

De igual forma, el factor s es un derivador. Es decir, la salida de ese sistema es la derivada de la entrada. La magnitud logarítmica de s=jω en decibeles es::

La pendiente m de la recta para jω es de:

El ángulo de fase del factor jω es constante e igual a 90°

La siguiente figura muestra curvas de respuesta en frecuencia para l/jω y jω, respectivamente.

Observar que ambas magnitudes logarítmicas se vuelven iguales a 0 dB en ω=1. (Cortan al eje de abcisas en ω=1)
Por tanto, si la función de transferencia contiene el factor (l/jω)n o (jω)n , la magnitud logarítmica se convierte, respectivamente, en:

Por tanto, las pendientes de las curvas de magnitud logarítmica para los factores (l/jω)n y (jω)n son -20n dB/década y 20n dB/década, respectivamente.
El ángulo de fase de (l/jω)n es igual a -90°n durante todo el rango de frecuencia, en tanto que el ángulo de fase de (jω)n es igual a 90°n en todo el rango de frecuencia. Las curvas de magnitud pasarán por el punto (0 dB, ω= 1).
Factores de primer orden . La magnitud logarítmica del factor de primer orden l/(1+jωT) en decibeles es:
. La magnitud logarítmica del factor de primer orden l/(1+jωT) en decibeles es:
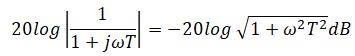
Para frecuencias bajas, tales que ω<<1/T, la magnitud logarítmica se aproxima mediante:

Por tanto, la curva de magnitud logarítmica para frecuencias bajas en este factor es la línea 0 dB constante. Para frecuencias altas, tales que :

Ésta última es una expresión aproximada para el rango de altas frecuencias. En ω=1/T , la magnitud logarítmica es igual a 0 dB; en ω=10/T, la magnitud logarítmica es de -20 dB. Por tanto, el valor de -20logωT dB disminuye en 20 dB para todas las décadas de ω. De esta forma, para ω>>1/T, la curva de magnitud logarítmica es una línea recta con una pendiente de -20 dB/década (o -6 dB/octava).
Nuestro análisis muestra que la representación logarítmica de la curva de respuesta en frecuencia del factor l/(1+jωT) se aproxima mediante dos asíntotas (líneas rectas), una de las cuales es una recta de 0 dB para el rango de frecuencia 0<ω<1/T y la otra es una recta con una pendiente de -20 dB/década (o -6 dB/octava) para el rango de frecuencia 1/T<ω<∞. La frecuencia en la cual las dos asíntotas se encuentran se denomina frecuencia de esquina o frecuencia de corte. Para el factor l/(1+jωT), la frecuencia ω=1/T es la frecuencia de esquina, dado que en ese punto ambas asíntotas tienen el mismo valor.

La función de transferencia de l/(1+jωT) tiene las características de un filtro pasa-baja. Para frecuencias por encima de ω=1/T, la magnitud logarítmica disminuye rápidamente hacia –infinito. Esto se debe, en esencia, a la presencia de la constante de tiempo. En el filtro pasa-baja, la salida sigue fielmente a la entrada sinusoidal a bajas frecuencias. Pero, conforme aumenta la frecuencia de entrada, la salida no puede seguir a la entrada debido a que se necesita cierta cantidad de tiempo para que el sistema aumente en magnitud. Por tanto, para altas frecuencias, la amplitud de la salida tiende a cero y el ángulo de fase tiende a -90°. En este aso, si la entrada posee muchos armónicos, las componentes de baja frecuencia se reproducen fielmente en la salida, mientras que las componentes de alta frecuencia se atenúan en amplitud y cambian en fase. Por tanto, un elemento de primer orden produce una duplicación exacta, o casi exacta, sólo para fenómenos constantes o que varían lentamente.
Resumen para polo simple:


Una ventaja de las trazas de Bode es que, para factores recíprocos, por ejemplo, el factor 1+jωT, las curvas de magnitud logarítmica y de ángulo de fase sólo necesitan cambiar de signo. Por tanto, la pendiente de la asíntota de alta frecuencia de 1+jωT es 20 dB/década, y el ángulo de fase varía de 0° a 90° conforme la frecuencia ω se incrementa de cero a infinito., como se puede ver en la siguiente Figura:

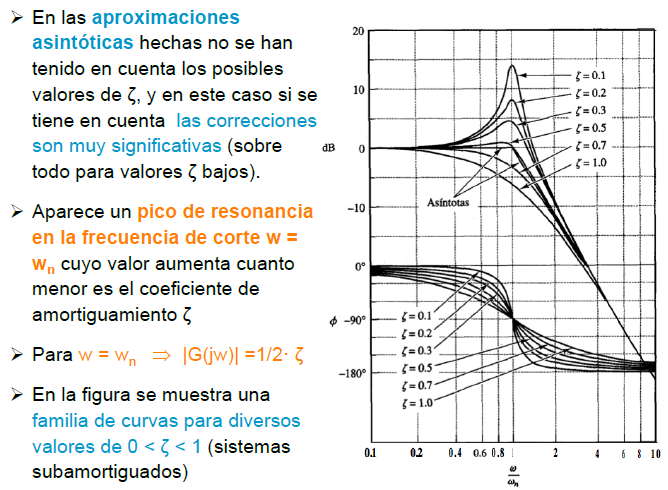
Se puede calcular el error en la curva de magnitud provocado por el uso de las asíntotas. El error máximo ocurre en la frecuencia de esquina y es aproximadamente igual a -3 dB debido a que:

El error es simétrico con respecto a la frecuencia de esquina. El error en decibelios implícito al usa la expresión asintótica para la curva de respuesta en frecuencia de l/(1+jωT) se muestra en figura siguiente:



CUADRÁTICOS
Factores cuadráticos . Los sistemas de control suelen tener factores cuadráticos de la forma:
. Los sistemas de control suelen tener factores cuadráticos de la forma:

Si ζ>1, este factor cuadrático se expresa como un producto de dos factores de primer orden con polos reales. Si 0<ζ<1, este factor cuadrático es el producto de dos factores complejos conjugados.
La curva asintótica de respuesta en frecuencia para  se obtiene del modo siguiente. Dado que:
se obtiene del modo siguiente. Dado que:

para frecuencias bajas tales que ω<<ωn, la magnitud logarítmica se convierte en:

Por tanto, la asíntota de frecuencia baja es una recta horizontal en 0 dB. Para frecuencias altas tales que ω>>ωn, la magnitud logarítmica se vuelve:

La ecuación para la asíntota de alta frecuencia es una recta con pendiente de -40dB/década, dado que:

La asíntota de alta frecuencia intercepta la de baja frecuencia en ω=ωn, dado que en esta frecuencia:

Esta frecuencia ωn es la frecuencia de esquina para el factor cuadrático considerado.





Ejemplo:
ANEXO 1.





ANEXO 2.

ANEXO 3.

ANEXO 4.


Te puede interesar:
Fuentes:
- Modern_Control_Engineering, Ogata 4t
- Control Systems Engineering, Nise
- Sistemas de Control Automatico, Kuo
Revisión literaria hecha por:
Prof. Larry Francis Obando – Technical Specialist – Educational Content Writer
Se hacen trabajos, se resuelven ejercicios!!
WhatsApp: +34633129287 Atención Inmediata!!
Copywriting, Content Marketing, Tesis, Monografías, Paper Académicos, White Papers (Español – Inglés)
Escuela de Ingeniería Electrónica de la Universidad Simón Bolívar, USB Valle de Sartenejas.
Escuela de Ingeniería Eléctrica de la Universidad Central de Venezuela, UCV CCs
Escuela de Turismo de la Universidad Simón Bolívar, Núcleo Litoral.
Contacto: España. +34633129287
Caracas, Quito, Guayaquil, Cuenca.
WhatsApp: +34633129287
FACEBOOK: DademuchConnection
email: dademuchconnection@gmail.com



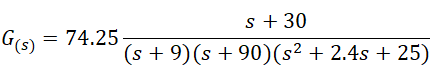




 ,
, ,
, .
.










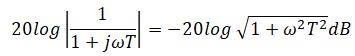











 se obtiene del modo siguiente. Dado que:
se obtiene del modo siguiente. Dado que:




































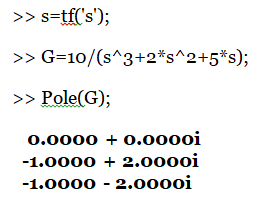












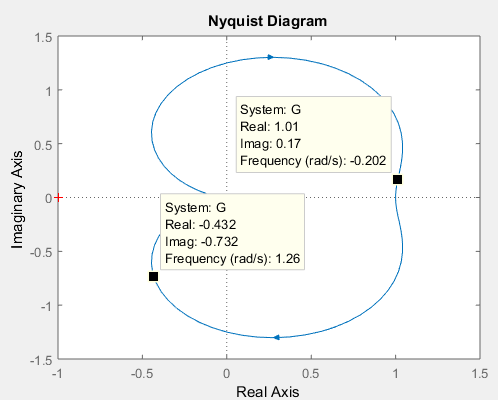
 la parte real se vuelve negativa. En
la parte real se vuelve negativa. En  , el diagrama de Nyquist cruza el eje real negativo ya que el término imaginario va a cero. El valor real en el cruce del eje, punto Q’ en la Figura 4 (c), es -0.874. Continuando hacia, la parte real es negativa, y la parte imaginaria es positiva. A frecuencia infinita:
, el diagrama de Nyquist cruza el eje real negativo ya que el término imaginario va a cero. El valor real en el cruce del eje, punto Q’ en la Figura 4 (c), es -0.874. Continuando hacia, la parte real es negativa, y la parte imaginaria es positiva. A frecuencia infinita: